Home » Geometry » Circle Calculator
Circle is part of our life and cannot be separated. Great Ancient invention wheel is in circular shape. In modern world GPS uses circles to calculating location on earth.
Calculate Circle and Sector Properties Area, Circumference and Circle’s Chord length here using our circle calculator online tool.
Basic parameters
| Diameter | 2 r | |
| Area | π r ² | |
| Perimeter | 2 π r |
Sector
| Arc Length | |
| Sector Area |
Chord
| Chord Length |
Circle is a simple shape formed by all points that are equal distance from a fixed point. The distance from center is called radius. The distance between two point on circle through center point is called diameter.
A constant ratio called pi(π) used in circle area and perimeter calculation. It is ratio of perimeter to diameter. Its value is 3.14159….
Equation x² + y² = r²
Circle drawn on triangle at end points or inside are incircle and circumcircle. See detailed explanation in centroid calculator.
Important definitions about circle
Basic Parameters
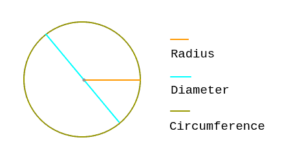
Radius
Distance between center of circle to any point of on circle.
Diameter
Distance between two points on circle line connecting them pass through center. It is two times of radius.
Circumference
Distance around the circle. Length of curve that drawn every point on curve is equidistant from a fixed point.
Sector
Part of Circle result of Division by angle at center. In the two of the divided parts, smaller one is minor sector and larger one is major sector. In the case of sector angle is 180 degrees the resultant sector is semi circle.
Lines over Circle
Chord
A line segment and its endpoints lie on the circle. The longest one is diameter.
Tangent
A straight line that pass though a single point of circle. Is perpendicular to radius line which has tangent line intersecting point on circle as endpoint.
Circle Properties
- Angle in a semi-circle is always 90 degrees.
- Perpendicular line from center divides a chord into two equal parts.
- Inscribed angle is always half of central angle for any two points on circle.
Solving chord length
Given angle at center or sagitta (length from center to middle point of chord).
With help of sagitta two right angle triangles formed. Then if angle is given, using trigonometry one can solve the chord length. In case of Sagitta is known, using Pythagoras theorem the chord length is solved.